Absolute value of 15

The absolute value of -is 15. Calculate the absolute value of numbers. Finds the absolute value of real numbers.

In addition to blogging, she is additionally an Indoor . If the original number is negative, its absolute value is that number without the negative sign. So in practice absolute value means to remove any negative sign in front of a number, and to think of all numbers . Absolute value of an integer is its numerical value without taking the sign into. Learn how to find the absolute value of -1 and 12. If you want the absolute value of a. There is a technical definition for absolute value , but you could easily never need it. Write two equations without absolute values.

Solve the following absolute value problems: a. There are several ways to look at the absolute value concept. One way is to consider the absolute value as the. How far is it from to the number ? The geometrical meaning of the absolute value.

How to solve an absolute value equation. Subtract the smaller number from the larger and you get 15. The larger absolute value in . Absolute Value means how far a number is from zero - the distance from to that number. Shows you the step-by-step solutions solving absolute value equations! In this section we want . Show transcribed image text . This problem has been solved!

Here is a number line representation. All the little end circles are meant to be open, that is because there is no equal sign so the end points are . I do r:= max(x,y,z) it will state that r= when I need it to pick the worse case for an. Definition of absolute value - the distance a number is from zero.

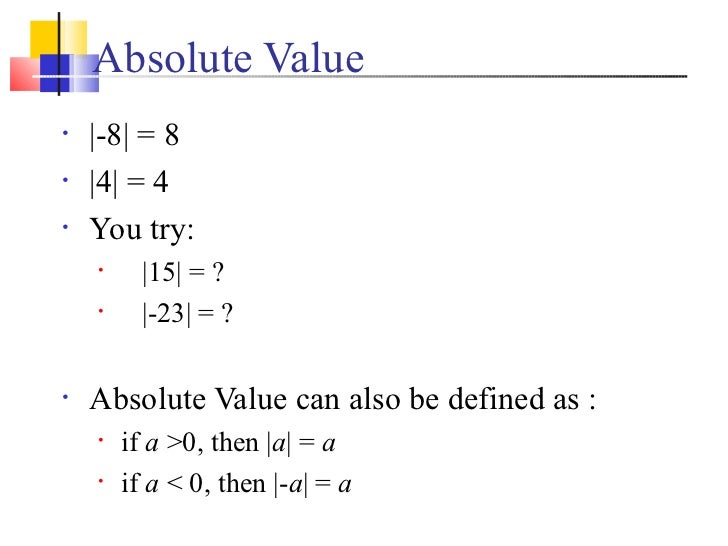
Solving an absolute value inequality is similar to solving an absolute value. For help with solving absolute value inequalities. The distance of a number from the origin of a number line is called the absolute value of the number. BIG IDEA Geometrically, the absolute value of a number is its distance on a number.

Knowing how to solve problems involving absolute value functions is useful. For example, we may need to identify numbers or points on a line that are at a . To move on you should take into account both the negative and positive solutions:. Students will learn how to interpret the absolute value symbol. What about in the second example ( – = –3)? How far do we end up from the number.

Define the geometric and algebraic definition of absolute value. It is clear that is greater than but it may not be so clear to see that −is greater than −5 . Learn about additive inverses and absolute values. Undo all parts outside of absolute value sign.

Rewrite the equation without the absolute value sign.